Parametric Equations
By: Carly Cantrell
Before we consider graphing in the polar plane, letÕs
understand the relationship between Cartesian and polar planes. Remember, in
the Cartesian plane y is a function of t, where t goes from 0 ˆ 2
. And in polar form, x is a function of t, where t
goes from 0 ˆ 2
and x is
the radial length, which is the horizontal distance from the origin.
Graph: y = cos(t) in the Cartesian plane
(left)
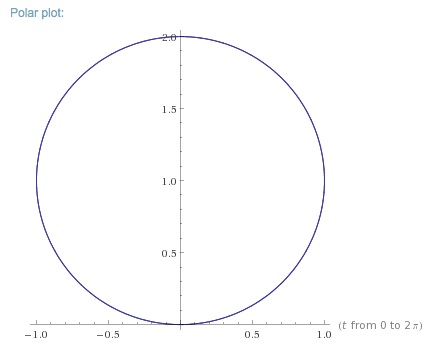
Graph: x = cos(t) in the polar plane
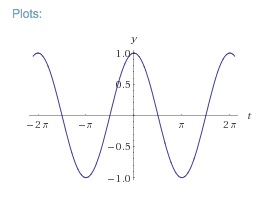
Notice, in both situations:
|
Input |
Output |
|
0 |
1 |
|
|
0 |
|
|
-1 |
|
3 |
0 |
|
2 |
1 |
The points are mapped in the same manner; they are just represented different based on the plane.
Parametric equations are graphed
in the polar plane.
Similarly,
Graph: y = sin(t) in the
Cartesian plane
(left)
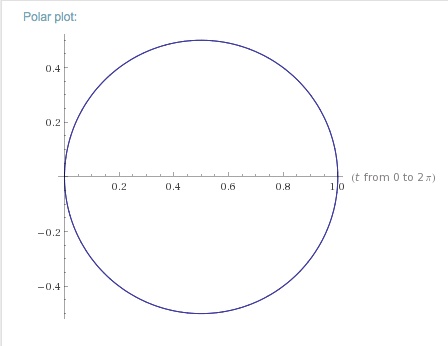
Graph: y = sin(t) in the
polar plane
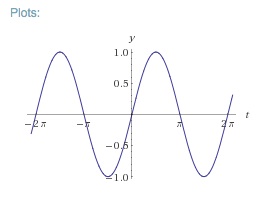
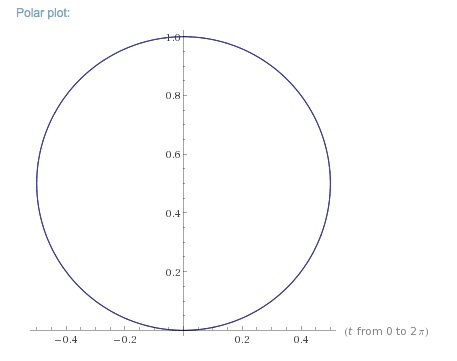
Now, in
polar form y represents the vertical distance away from the origin and the
following table represents both graphs:
|
Input |
Output |
|
0 |
0 |
|
|
1 |
|
|
0 |
|
3 |
-1 |
|
2 |
0 |
LetÕs investigate the results when we plug in various
a and b in the forms:
x = cos(at)
y = sin(bt)
for 0 
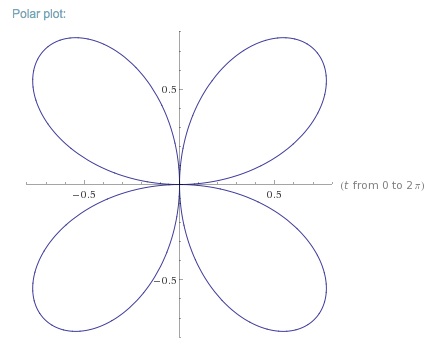
When a and b are both 2:
x = cos(2t)
y =
sin(2t)
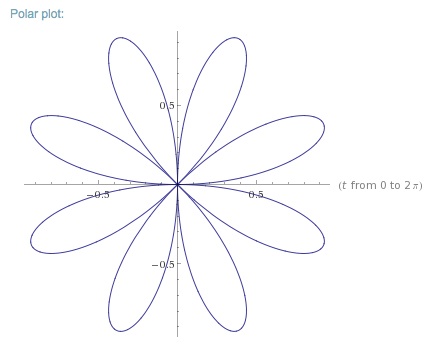
When a and b are both 3:
x = cos(3t)
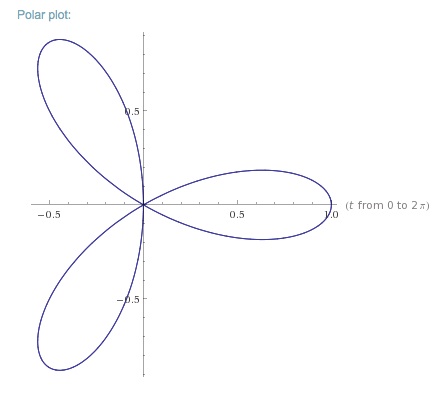
y =
sin(3t)
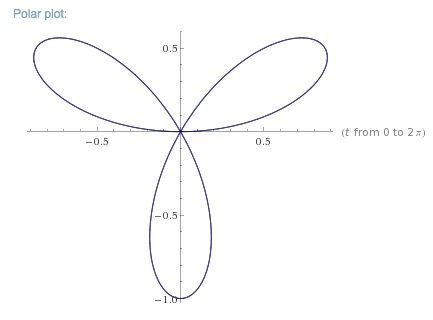
When a and b are both 4:
x = cos(4t)
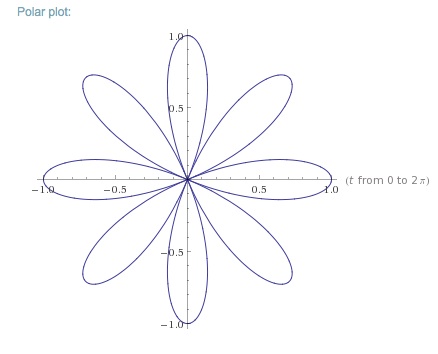
y =
sin(4t)
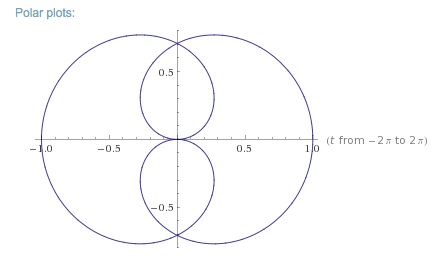
When and b are both
:
Question: How come for even integers there are twice
as many petals as the integer, but for odd integers there are the same number
of petals as the integer?
Answer: When there are even integers, from 0 ˆ
2
, there is no
overlap. Whereas, when mapping with an odd integer, there is overlap. This
means from 0 ˆ 
and from 
ˆ 2
the same
points are being swept by those angle measures.
Conjecture: When the integer is less than one, the
graphs are identical! Investigate for yourself!
LetÕs investigate the results when we plug in various
a and b in the forms:
x = acos(t)
y = bsin(t)
for 0 
This results in a radial scaling away from the origin.
x =
2cos(t)
y =
2sin(t)
